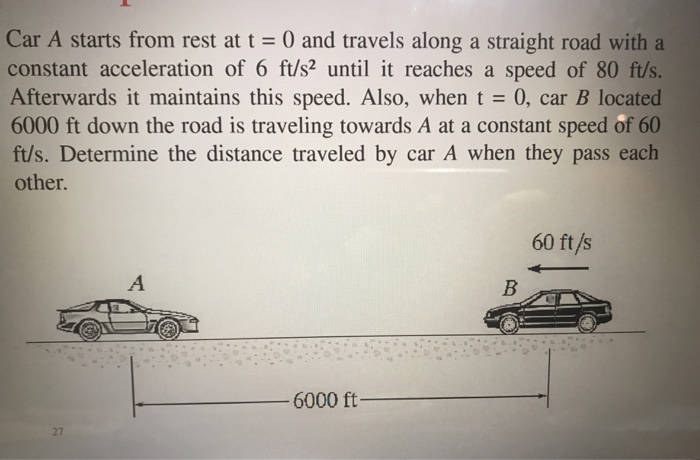
I saw the detailed answer for the question, but I didn't understand the computations and visualize it.

Ans:3200ft

Show transcribed image text

Expert Answer

Was this answer helpful?

---

General guidance

Concepts and reason

The concepts required to solve the given problem are kinematic equations of motion.

Initially, calculate the distances travelled by the cars A and B by using kinematic equations of motion. Next, calculate the distance between cars by using the total distance. After that, calculate the relative velocity of the cars by using the velocities of car A and car B. Finally, calculate the distance travelled by car A when they pass each other by using the distance travelled by the cars A and B.

Fundamentals

The kinematic equations of motion are,

$\begin{array}{l}\\v = u + at\\\\s = ut + \frac{1}{2}a{t^2}\\\end{array}$

Here, u is the initial velocity, v is the final velocity, s is the distance, a is the acceleration, and t is the time taken.

Show more 

FIRST STEP | ALL STEPS | ANSWER ONLY

Step-by-step

Step 1 of 2

The time taken for the car A is,

$t = \frac{{v - u}}{a}$

Since the car A is initially at rest. So, the initial velocity is zero.

$u = 0\;{\rm{ft/s}}$

Substitute 80 ft/s for v, 0 m/s for u, and $6\;{\rm{ft/}}{{\rm{s}}^2}$ for a in the above equation.

$\begin{array}{c}\\t = \frac{{80\;{\rm{ft/s}} - 0\;{\rm{ft/s}}}}{{6\;{\rm{ft/}}{{\rm{s}}^2}}}\\\\ = 13.3333\;{\rm{s}}\\\end{array}$

The distance travelled by the car A is,

${s_{{\rm{A1}}}} = {u_{\rm{A}}}t + \frac{1}{2}{a_{\rm{A}}}{t^2}$

Substitute 0 for ${u_{\rm{A}}}$ , 13.3333 s for t, and $6\;{\rm{ft/}}{{\rm{s}}^2}$ for ${a_{\rm{A}}}$ in the above equation.

$\begin{array}{c}\\{s_{{\rm{A1}}}} = \left( 0 \right)\left( {13.3333\;{\rm{s}}} \right) + \frac{1}{2}\left( {6\;{\rm{ft/}}{{\rm{s}}^2}} \right){\left( {13.3333\;{\rm{s}}} \right)^2}\\\\ = \frac{1}{2}\left( {6\;{\rm{ft/}}{{\rm{s}}^2}} \right){\left( {13.3333\;{\rm{s}}} \right)^2}\\\\ = 533.33\;{\rm{ft}}\\\end{array}$

The distance travelled by car B towards the car A is,

${s_{\rm{B}}} = {u_{\rm{B}}}t + \frac{1}{2}{a_{\rm{B}}}{t^2}$

Substitute 60 ft/s for ${u_{\rm{B}}}$ , 13.3333 s for t, and 0 for ${a_{\rm{B}}}$ in the above equation.

$\begin{array}{c}\\{s_{\rm{B}}} = \left( {60\;{\rm{ft/s}}} \right)\left( {13.3333\;{\rm{s}}} \right) + \frac{1}{2}\left( 0 \right){\left( {13.3333\;{\rm{s}}} \right)^2}\\\\ = \left( {60\;{\rm{ft/s}}} \right)\left( {13.3333\;{\rm{s}}} \right)\\\\ = 799.99\;{\rm{ft}}\\\end{array}$

If car B has constant speed, then the acceleration of the car B is equal to zero.

Explanation | Common mistakes | Hint for next step

The first kinematic equation of motion is,

$v = u + at$

Rearrange the above equation for the time.

$\begin{array}{c}\\v - u = at\\\\t = \frac{{v - u}}{a}\\\end{array}$

Step 2 of 2

Since the cars started at the distance 6000 ft. At 13.33 s, the cars separated by the distance is ${s_{{\rm{AB}}}}$ .

The distance between the cars A and B is,

${s_{{\rm{AB}}}} = {s_{{\rm{total}}}} - \left( {{s_{{\rm{A}}1}} + {s_{\rm{B}}}} \right)$

Here, ${s_{{\rm{total}}}}$ is the starting distance of the cars.

Substitute 533.33 ft for ${s_{{\rm{A}}1}}$ , 6000 ft for s, and 799.99 ft for ${s_{\rm{B}}}$ in the above equation.

$\begin{array}{c}\\{s_{{\rm{AB}}}} = 6000\;{\rm{ft}} - \left( {533.33\;{\rm{ft + 799}}{\rm{.99}}\;{\rm{ft}}} \right)\\\\ = 4666.68\;{\rm{ft}}\\\end{array}$

The total time taken by the car A to cross the car B is,

$\begin{array}{c}\\{v_{{\rm{rel}}}} = \frac{{{s_{{\rm{AB}}}}}}{{{t_{\rm{A}}}}}\\\\{t_{\rm{A}}} = \frac{{{s_{{\rm{AB}}}}}}{{{v_{{\rm{rel}}}}}}\\\end{array}$

Substitute 4666.68 ft for ${s_{{\rm{AB}}}}$ and 140 ft/s for ${v_{{\rm{rel}}}}$ in the above equation.

$\begin{array}{c}\\{t_{\rm{A}}} = \frac{{4666.68\;{\rm{ft}}}}{{140\;{\rm{ft/s}}}}\\\\ = 33.33\;{\rm{s}}\\\end{array}$

So, the distance travelled by the car A is,

${s_{{\rm{A2}}}} = {v_{\rm{A}}}{t_{\rm{A}}}$

Substitute 80 ft/s for ${v_{\rm{A}}}$ and 33.33 s for ${t_{\rm{A}}}$ in the above equation.

$\begin{array}{c}\\{s_{{\rm{A2}}}} = \left( {80\;{\rm{ft/s}}} \right)\left( {33.33\;{\rm{s}}} \right)\\\\ = 2666.67\;{\rm{ft}}\\\end{array}$

The distance travelled by the car A when they pass each other is,

${s_{\rm{A}}} = {s_{{\rm{A1}}}} + {s_{{\rm{A2}}}}$

Substitute 533.33 ft for ${s_{{\rm{A1}}}}$ and 2666.67 ft for ${s_{{\rm{A2}}}}$ in the above equation.

$\begin{array}{c}\\{s_{\rm{A}}} = 533.33\;{\rm{ft + }}2666.67\;{\rm{ft}}\\\\{\rm{ = 3199}}{\rm{.99}}\;{\rm{ft}}\\\\ \simeq 3200\;{\rm{ft}}\\\end{array}$

The distance travelled by the car A when they pass each other is 3200 ft.

Explanation | Common mistakes

Since the total distance is 6000 ft.

$\begin{array}{c}\\{s_{\rm{A}}} + {s_{\rm{B}}} + s = 6000\;{\rm{ft}}\\\\s{\rm{ = }}6000\;{\rm{ft}} - {s_{\rm{A}}} - {s_{\rm{B}}}\\\\ = 6000\;{\rm{ft}} - \left( {{s_{\rm{A}}} + {s_{\rm{B}}}} \right)\\\end{array}$

If the cars are moving in opposite direction, the relative speed between the cars A and B is,

${v_{{\rm{rel}}}} = {v_{\rm{A}}} - {v_{\rm{B}}}$

Substitute -60 ft/s for ${v_{\rm{B}}}$ and 80 ft/s for ${v_{\rm{A}}}$ in the above equation.

$\begin{array}{c}\\{v_{{\rm{rel}}}} = 80\;{\rm{ft/s}} - \left( { - 60\;{\rm{ft/s}}} \right)\\\\ = 80\;{\rm{ft/s + 60}}\;{\rm{ft/s}}\\\\{\rm{ = 140}}\;{\rm{ft/s}}\\\end{array}$

Since the car B is moving opposite direction. So, the velocity of car B is negative.

Answer

The distance travelled by the car A when they pass each other is 3200 ft.