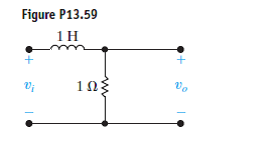
Problem
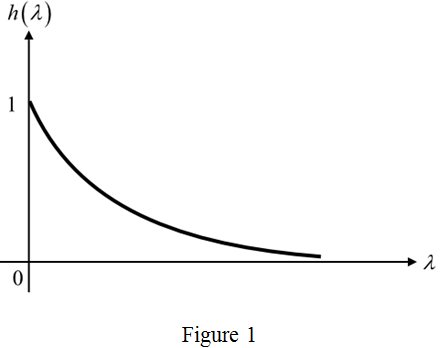
A rectangular voltage pulse vi = [u(t) - u(t - 1)] V is applied to the circuit in Fig. P13.59. Use the convolution integral to find vo.
Step-by-step solution
Step 1 of 8
Refer to Figure P13.59 in the textbook for the circuit diagram.
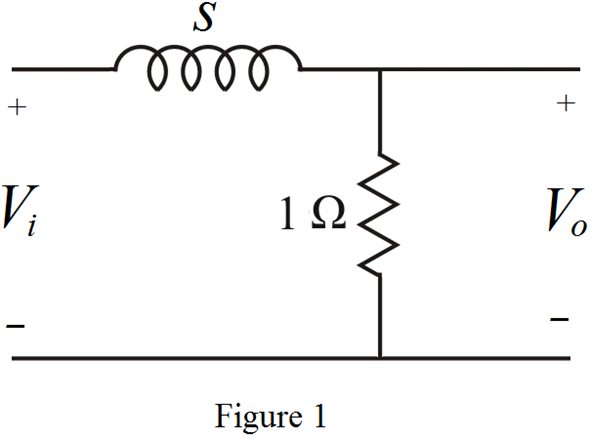
The s-domain representation of the circuit is shown in Figure 1.
Comment Step 2 of 8
Calculate the output voltage using voltage division rule.
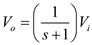
The transfer function is,

Apply inverse Laplace transform on both sides.

Replace
 with
with 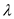 .
.
Here, the impulse response is a decaying exponential. So, plot the impulse function.
Comment Step 3 of 8
Consider the input voltage,
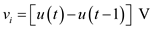 . Replace
. Replace  with
with 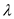 .
.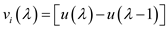
The mathematical representation is,
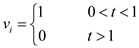
Plot the input signal.

Comment Step 4 of 8
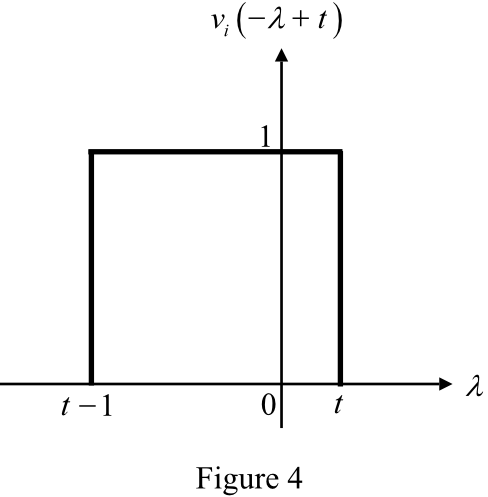
The folded excitation function
 is shown in Figure 3.
is shown in Figure 3.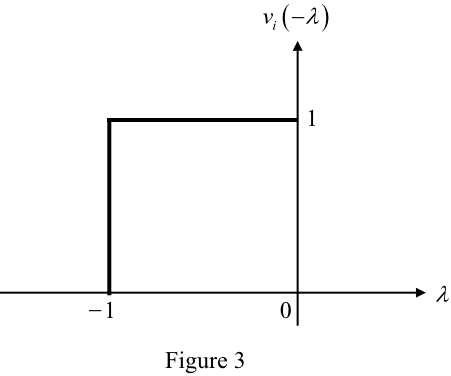
The convolution integral is,

Plot the signal,
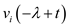 .
.
Comment Step 5 of 8
Apply the convolution to both Figure 4 and Figure 1.
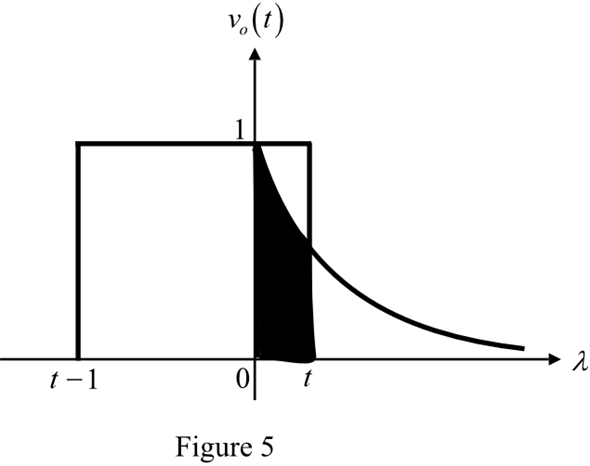
Comment Step 6 of 8
From Figure 5, the output voltage in the interval of
 is,
is,
Comment Step 7 of 8
Now apply the convolution to both Figure 4 (when
 ) and Figure 1.
) and Figure 1.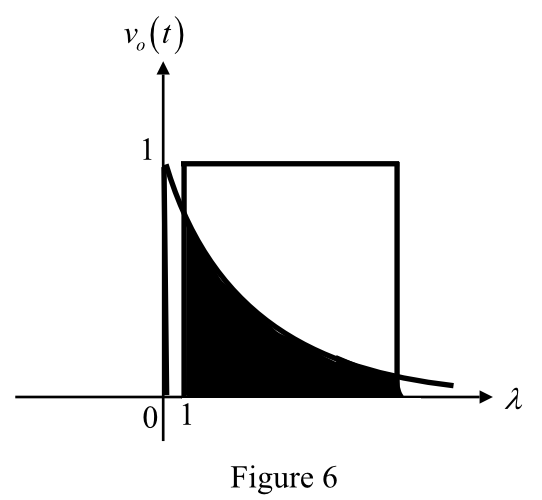
Comment Step 8 of 8
From Figure 5, the output voltage in the interval of
 is,
is,
The output waveform is shown in Figure 7.
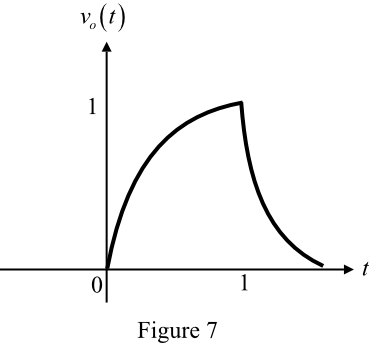
Hence, the output has been obtained.
Comments (1)
